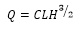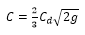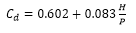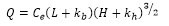Written by Christopher Goodell, P.E., D.WRE
Copyright © The RAS Solution 2016. All rights reserved.
HEC-RAS has the ability to simulate flow at hydraulic
controls in a variety of ways.
Bridges, culverts, inline structures, lateral structures, and SA/2D area connections can
all act as hydraulic controls.
Effectively, they break up the conservation equations used between cross
sections in a 1D reach and/or cells in a 2D area with empirically derived (and usually
very stable!) equations. Weir equations
can be used to define flow over an obstruction and are available with all of
the 5 hydraulic controls identified above.
However, there are a number of options to consider when selecting
simulating weir flow in HEC-RAS. HEC-RAS
approaches weir flow with three different cases:
Ungated Inline Weirs, Ungated Lateral Weirs, and Gated Weirs. They all begin with the same standard equation:
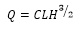
(1)
Where:
Q = discharge, C =weir coefficient, L = weir crest length, H = Energy
head over the weir crest.
But each of the three cases apply the weir equation slightly
differently.
Before I continue, I should discuss the
difference between the weir coefficient and the discharge coefficient. I see both of them used interchangeably, but
they ARE different. The weir coefficient
(as shown above in the weir equation) is a lumped parameter that includes the
discharge coefficient, the gravitational constant, and constants based on
geometric properties.
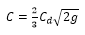
(2)
Where Cd is the discharge
coefficient.
The discharge coefficient
is dimensionless and therefore it is the same in both English (U.S. Customary)
Units and SI Units. The weir
coefficient, since it is a function of the gravitational constant, is not
dimensionless and therefore has different values depending on which unit system
you are using. For example, a weir
coefficient (C) of 3.00 in English Units would be 1.66 in SI units. But both share the same discharge coefficient
(Cd) of 0.56. For convenience, to
convert an English weir coefficient to an equivalent SI weir coefficient,
multiply the English weir coefficient by 0.552.
Be very cautious when considering C
versus Cd. They are different but are
often mistakenly used interchangeably.
In fact, you’ll see the coefficient Cd labeled occasionally in the
HEC-RAS software and literature when discussing weir coefficient.
Ungated Inline Weirs.
When defining inline flow over an “ungated” obstruction
(bridge, culvert embankment, inline structure, SA/2D area connection), you have
two options for computing weir flow:
Broad Crested and Ogee.
Figure 1. Inline structure weir embankment editor.
Both use the same standard weir equation presented above in
equation (1).
The only difference between the Broad
Crested Option and the Ogee Option is that for the Broad Crested option, the
user enters a weir coefficient for C.
For the Ogee option, the user enters a spillway approach height and the
ogee’s design energy head, and HEC-RAS will compute the weir coefficient for
you. This may sound convenient, but as
the name implies, this option should really be used only for ogee-shaped
spillways. And you would have to know
what the design energy head is, a design parameter that is not usually easy to
come by, unless you have the hydraulic design report for the spillway. With both options, submergence reduction of
the discharge is automatically calculated with their own respective methods
(FHWA ,1978 for broad crested, and COE, 1965 for ogee).
Ungated Lateral Weirs.
Lateral weirs are entered in the lateral
structure editor. Inside the lateral
structure’s weir embankment editor, you’ll see two options for weir
computations: Standard Weir Eqn. and
Hager’s Eqn.
Figure 2. Lateral
Weir Embankment Editor.
In version 5.0.1, the Standard Weir Eqn.
provides four options for the weir crest shape:
Broad Crested, Ogee, Sharp Crested, and Zero Height. Caution! Zero Height is NOT used when Standard Weir
Eqn. is selected. This is a bug and
will most likely be fixed for future versions.
If you do select Zero Height and Standard Weir Eqn. together, HEC-RAS
will just use the weir coefficient you provide with the broad crested
methodology. Sharp Crested is not fully
functional in Lateral Structures for version 5.0.1. You’ll notice that no additional input
options (like Rehbock and Kindsvater-Carter, as discussed under the next
section, “Gated Weirs) are available when you select Sharp Crested in the
lateral weir embankment editor. My guess
is that if you select Sharp Crested, it too will default to the broad crested methodology.
Broad Crested and Ogee work the same as
with the ungated inline structures.
With Hager’s Equation, all four weir
crest shapes are available, including the zero-height weir. The same weir equation is used, but an
adjusted weir coefficient is computed based on physical and hydraulic
properties. Each of the four weir types
has its own method for computing the adjusted weir coefficient. There is an input box for “default weir
coefficient”. This is only used for the
first iteration of solving Hager’s Equation.
Since Hager is a function of hydraulic properties, it must be solved in an
iterative fashion. After the first
iteration, the adjusted weir coefficient will be computed and used. Page 8-18 of the Hydraulic reference manual
discusses Hager’s equation and how the adjusted weir coefficient is
computed.
Zero-height weirs are used for cases
where flow will leave a channel laterally, but there is no defined obstruction
or hydraulic control separating the two.
Commonly this is used to simulate flow from a main channel up a
tributary that is being modeled using a lateral structure and a storage or 2D
area. The HEC-RAS 2D manual has a table
of lateral weir coefficients (Table 1).
Table 1. Lateral Weir Coefficients (from the HEC-RAS
2D Manual, page 3-50).
Notice the last category is “non elevated”
overbank terrain. If you wish to use the
weir coefficients in this table to simulate a non-elevated weir, do not use
the Zero-Height weir. That is
strictly for Hager’s equation and Hager’s method automatically computes the
weir coefficient. Instead, use the broad
crested standard equation and enter in the non-elevated weir coefficient
there.
Gated Weirs.
When modeling gated spillways at inline
structures or lateral structures, users can provide a weir coefficient for flow
over the spillway when the gate is completely opened, and out of contact with
the flow (Figure 3). This is different from the discharge coefficient used for
flow over the top of the inline structure (Figure 1).
Figure 3.
Inline Gate Editor
With gated spillways, the user has three
options for the weir shape: Broad
Crested, Sharp Crested, and Ogee (Figure 4).
Broad Crested and Ogee work the same as previously discussed. The Sharp Crested option also uses the
standard weir equation but gives you three options for determining the
discharge coefficient: user-entered,
compute with the Rehbock equation, or compute with the Kindsvater-Carter
equation. For both the Rehbock and
Kinsvater-Carter methods, the weir coefficient will be computed independently
at each time step. So you can have a varying discharge coefficient for varying
heads.
Figure 4. Inline Gate Editor.
The Rehbock equation for the discharge
coefficient was developed for rectangular weirs and is as follows:
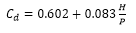
(3)
Where P = Spillway approach height. This value must be entered to use the Rehbock
equation. HEC-RAS will then compute the
weir coefficient, C using equation (2).
According to Ippen (1950), this equation holds up well for values of H/P
up to 5. And it performs with fair
approximation for H/P values up to 10.
The Kindsvater-Carter method was
developed in English units only and is as follows:
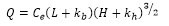
(4)
Where Ce = effective weir coefficient, ft1/2/s
kb
= a correction factor to obtain effective weir crest length, ft
kh
= a correction factor with a constant value of 0.003 ft
The effective weir coefficient, Ce is a function of two
ratios: L/B and H/P,
Where L = Weir crest length
B = Average width of the approach channel
H = Energy head over the weir crest
P = Spillway approach height
Ce is a function of both the relative width and
relative depth of the approach channel and is taken from the following chart
(note that the chart uses the variable h1 for H. They are the same):
Figure 5. Effective Weir Coefficient
kb is used to determine the
effective length of the weir crest and is a function of the relative width of
the approach channel. It is taken from
the following chart:
Figure 6. Correction factor kb.
To use the Kindsvater-Carter method in
HEC-RAS for a gated spillway, first select the weir shape as “Sharp
Crested”. Then select “Compute with
Kinsvater-Carter eqn as the Weir Method.
You must then choose a relative approach channel width (L/b) and enter
the spillway approach height, P (note, b is used in the HEC-RAS Inline Gate
Editor for B. They are the same).
Figure 7. Kindsvater-Carter Weir Method.
Remember, the Kindsvater-Carter equation
was developed and is presented here in English units. When using SI units, HEC-RAS will
automatically convert the units appropriately.
So you can still enter a spillway approach height in meters if you are
using SI units.
The Kindsvater-Carter weir equation is
built for rectangular weirs and “is particularly useful for installations where
full crest contractions or full end contractions are difficult to
achieve.” (USBR 2001) More information on the Kindsvater-Carter
equation, including its limitations, can be found here:
http://www.usbr.gov/tsc/techreferences/mands/wmm/chap07_06.html
References:
Federal Highway Administration (FHWA),
1978. Hydraulics of Bridge Waterways, Hydraulic Design Series No. 1, by
Joseph N. Bradley, U.S. Department of Transportation, Second Edition, revised
March 1978, Washington D.C.
Ippen, A.T. ,1950. Channel Transitions and Controls, Chap. VIII
in Hunter Rouse (editor): Engineering Hydraulics,” John Wiley & Sons, Inc.,
New York. pp.496-588.
U.S. Army Corps of Engineers (COE),
1965. Hydraulic Design of Spillways, EM 1110-2-1603, Plate 33.
Over the two days there
will be plenty of opportunities to speak to Chris, Gary and Matt and learn from
the experts. Demonstrations and interactive workshops will feature the latest
thinking, giving you practical solutions to take away
with you.
